Linear Approximations and Tangent Planes

University
Massachusetts Institute of Technology
Course
Multivariable Calculus
Pages

2

Academic year

2022
Author

Sporkz
Views

31

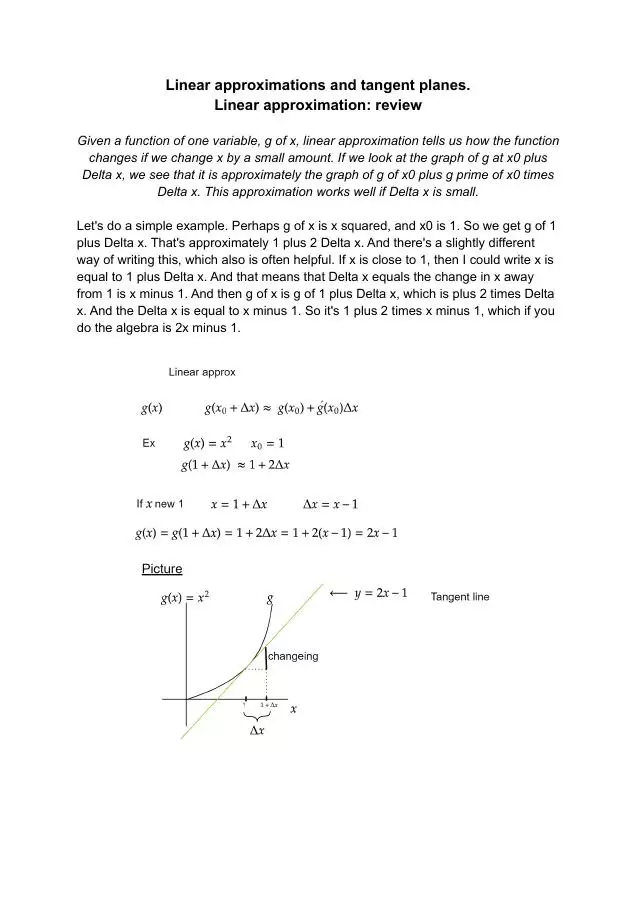
Linear approximations and tangent planes. Linear approximation: review Given a function of one variable, g of x, linear approximation tells us how the function changes if we change x by a small amount. If we look at the graph of g at x0 plus Delta x, we see that it is approximately the graph of g of x0 plus g prime of x0 times Delta x. This approximation works well if Delta x is small. Let's do a simple example. Perhaps g of x is x squared, and x0 is 1. So we get g of 1plus Delta x. That's approximately 1 plus 2 Delta x. And there's a slightly differentway of writing this, which also is often helpful. If x is close to 1, then I could write x isequal to 1 plus Delta x. And that means that Delta x equals the change in x awayfrom 1 is x minus 1. And then g of x is g of 1 plus Delta x, which is plus 2 times Deltax. And the Delta x is equal to x minus 1. So it's 1 plus 2 times x minus 1, which if youdo the algebra is 2x minus 1.

Here is the function g of x. Here is the derivative of g, which is simply 2x squared.And what is the linear approximation? One thing we could write is g is equal to twotimes x minus one.So it looks like that. So here in white is the actual function g,andin green is y equals two times x minus one. If we wanted to consider the 1 + Delta x version of our equation, we could substitute1 + Delta x for g in our original equation. The linear approximation is a function that describes how the curve changes as thevalue of x approaches zero. The main point to remember is that when Delta x issmall, the green line and white curve are nearly identical. This means that whenDelta x is small, the linear approximation is accurate.When Delta is large, the linear approximation is not very good. And you can think ofit as the line that hugs the curve as closely as possible. And because it's trying tohug the curve as closely as possible, it's tangent to the curve. So this here is atangent line.